Math
Exercise 12
Choose the best answer.
Find the Derivative:
1. ![]()
(a) |
|
(b) |
|
(c) |
|
(d) |
(b) ![]()
![]()
2. x2 cos x.
(a) |
-2x sin x |
(b) |
2x cos x + x2 sin x |
(c) |
2x cos x – x2 sin x |
(d) |
2x – sin x |
(c) 2x cos x – x2 sin x
2x cos x + x2(-sin x) = 2x cos x - x2sin
3. ![]()
(a) |
e2x |
(b) |
|
(c) |
2x ln x2 |
(d) |
2ex |
(b) ![]()
Use Rule 10: e to the power of x2 • 2x
4. loga x
(a) |
1/(x ln a) |
(b) |
1/x |
(c) |
|
(d) |
|
(a) 1/(x ln a)
Use this rule: (loga x)1 = 1 / (x ln a)
5. ![]()
(a) |
(b) |
|
|
(c) |
(d) |
(d) ![]()
Use Rules 6 and 9.
6. sin (x3 + 3x2 + 1)
(a) |
cos(x3 + 3x2 + 1) + (3x2 + 6x) |
(b) |
(3x2 + 6x) cos(x3 + 3x2 + 1) |
(c) |
(3x2 + 6x) (-cos(x3 + 3x2 + 1)) |
(d) |
cos(3x2 + 6x) |
(b) (3x2 + 6x) cos(x3 + 3x2 + 1)
Rule 10: cos (x3 + 3x2 + 1) • 3x2 + 6x
Note: derivative of a constant is zero.
7. ![]()
(a) |
(b) |
|
|
(c) |
(d) |
(c) ![]()
x –½
so derivative is: - ½ x –1½
8. cos x tan x
(a) |
cos x |
(b) |
tan x |
(c) |
-sin x sec2 x |
(d) |
|
(a) cos x
– sin x • tan x + cos x/cos2x = – sin x • sin x/cos x + 1/cos x
Now combine with this rule: sin2x + cos2x = 1
cos2x/cos x = cos x
9. ![]()
(a) |
(b) |
|
|
(c) |
(d) |
(d) ![]()
Use Rule 10: -2 (3x – 4)-3 • 3
10. Let the position function x(t) = 100t – 5t2represent the location of a moving particle. When t = 0 sec., x = 0 feet.
i) What is the average speed (in feet per second) of the car between t = 1 and t = 2.
(a) |
80 |
(b) |
85 |
(c) |
90 |
(d) |
180 |
(b) 85
calculate position at t = 1 and t =2.
The difference is the change in location in one second, which is the average speed.
ii) What is the instantaneous speed (in feet per second) at t = 2.
(a) |
80 |
(b) |
90 |
(c) |
100 |
(d) |
180 |
(a) 80
v (2) = x’(2) = 100 – 10t = 80
11. A biologist has estimated that if a bactericide is introduced into a culture of bacteria, the number of bacteria, B(t), present at time t (in hours) is given by B(t) = 1000 + 50t – 5t2 million.
i) Find the instantaneous rate of change of the number of bacteria with respect to time.
(a) |
|
(b) |
1050 – 10t |
(c) |
50t – 10t |
(d) |
50 – 10t |
(d) 50 – 10t
B’(t) = rate of change (t) = 50-10t (million/hour) 13
ii) Find the instantaneous rate of change of the number of bacteria with respect to time (in millions per hour) when t = 3 hours.
(a) |
10 |
(b) |
20 |
(c) |
120 |
(d) |
1020 |
(b) 20
At t = 3: 50 - 10 • 3 = 20 million/hr
iii) After how many hours does the population of bacteria start to decline?
(a) |
1 |
(b) |
5 |
(c) |
10 |
(d) |
12 |
(b) 5
“start to decline” means that the rate of change = 0, so 50 – 10 t = 0
iv) What is the maximum population of the bacteria (in millions)?
(a) |
1125 |
(b) |
1500 |
(c) |
1700 |
(d) |
2100 |
(a) 1125
At t = 5, population is max, so B(5) = 1000 + 50t – 5 t2 = 1000 + 50(5) – 5 (5)2
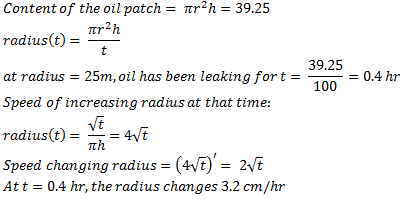
12. Suppose thick oil is leaking from a submerged wreck at the rate of 100 litres per hour. The oil forms a circular patch on the surface with a constant thickness of 2 cm. How fast is the radius of the oil patch increasing (in centimetres per hour) when the radius is 25 m?
(a) |
0.02 | (b) |
1.2 |
(c) |
2.1 | (d) |
3.2 |
(b) 3.2
13. f (a) is a relative maximum value for the function f (x) when:
(a) |
f ' (a) = 0 and f '' (x) > 0 |
(b) |
|
(c) |
|
(d) |
f ' (a) = 0 and f '' (a) = 0 |
(b) ![]()
Consider a ball you throw in the air: first the ball goes up (f(x)), has a max (f(a)), and comes down.
Vertical speed of ball is positive when going up, becomes zero at top and increases when coming down (negative). (f’(x))
Acceleration decreases, becomes zero and increases. (f’’(x))
14. Find the x-coordinate of the relative maxima and relative minima (if they exist) of ![]()
(a) |
x = -1 |
(b) |
x = +1 |
(c) |
x = ½ |
(d) |
no relative maxima or minima for function |
(d) no relative maxima or minima for function
f’(x) = (x + 1)-2
f’’(x) = -2 (x + 1)-3
maxima: f(a) = max, f’(a) = 0, f’’(x) = 0
and x<a => f’(x) >0, x>a => f’(x) <0
minima: f(a) = min, f’(x) = 0, f’’(x) = 0
and x>a => f’(x) >0, x<a => f’(x) <0
Solving for these equation, you 1/ [(x-1) (x+1)] = 0. There are no solutions for this.
15. A biologist has found that the percent of selenium in the soil x months after flushing the area with clean water is given by ![]() , 1 ≤ x ≤ 12. When (in months) will the selenium be reduced to a minimum?
, 1 ≤ x ≤ 12. When (in months) will the selenium be reduced to a minimum?
(a) |
30 |
(b) |
5 |
(c) |
12 |
(d) |
1 |
(b) 5
Minimum when speed with which selenium disappears from soil is zero.
So we look for f’(x) = 0
f’(x) = (x2 – 36)/ 2x2 = 0
This will only happen if x2 – 36 = 0, so x = -6 or x = + 6. Only 6 is a logical solution here.