Math
Vectors
Consider a point P at (x, y). The vector ![]() , represented by an arrow extending from the origin to P is represented as (x , y). The magnitude of the vector is the length of the arrow and the direction is the angle it makes with one of the axis. In 3 dimensions we add a third coordinate and get a point P(x, y, z) and the corresponding vector
, represented by an arrow extending from the origin to P is represented as (x , y). The magnitude of the vector is the length of the arrow and the direction is the angle it makes with one of the axis. In 3 dimensions we add a third coordinate and get a point P(x, y, z) and the corresponding vector ![]() .
.
If we have vectors ![]() and
and ![]() the sum
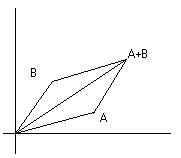
the sum ![]() defined to be (3 + 1, 2 + 4) or (4, 6). Recall the parallelogram law.
defined to be (3 + 1, 2 + 4) or (4, 6). Recall the parallelogram law.
In general if ![]() and
and ![]() then
then ![]()
or in three dimensions ![]()
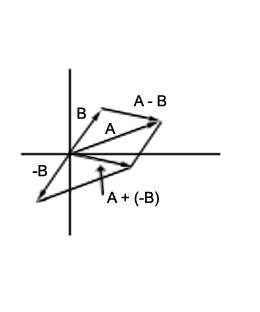
If the vector ![]() then the vector
then the vector ![]() (same magnitude, opposite direction). We can therefore define the difference of vectors.
(same magnitude, opposite direction). We can therefore define the difference of vectors.
![]()
If vector A is ![]() and B is
and B is ![]() then
then ![]()
For example let ![]() and
and ![]() . Then
. Then ![]() or
or ![]()
In three dimensions we have: If ![]() and
and ![]() then
then 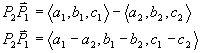
The magnitude of a vector is the length of the arrow. In two dimensions the magnitude of ![]() . In three dimensions the magnitude of
. In three dimensions the magnitude of ![]() .
.
Frequently it is useful to use unit vectors or vectors of magnitude 1. The basic unit vectors in three dimensions are i = (1, 0, 0), j = (0, 1, 0) and k = (0, 0, 1). Using this notation the vector (a, b, c) can be also written ai + bj + ck.